APEX Antenna Efficiencies
| 2017 | 2018 | 2019 | 2020 | 2021 | 2022 | 2023 | 2024 |
|---|
| Mars | Jupiter | Uranus | All |
|---|
| Instrument | Year-Month | Planet | θS ('')1 | Frequency (GHz) | θmb ('') | ηa2,4 | ηmb3,4 | Jy/K5 | # Scans |
|---|---|---|---|---|---|---|---|---|---|
| NFLASH230 | 2020-09 | MARS | 22.2 ± 0.16 | 204.83 | 29.5 | 0.59 ± 0.05 | 0.72 ± 0.06 | 39 ± 3 | 8 |
| NFLASH230 | 2020-09 | MARS | 20.7 ± 0.01 | 230.37 | 26.3 | 0.55 ± 0.05 | 0.68 ± 0.06 | 42 ± 3 | 6 |
| PI230 | 2020-09 | MARS | 21.4 ± 0.01 | 230.54 | 26.3 | 0.59 ± 0.05 | 0.72 ± 0.06 | 40 ± 3 | 8 |
| PI230 | 2020-09 | JUPITER | 42.0 ± 0.00 | 230.54 | 26.3 | 0.64 ± 0.06 | 0.79 ± 0.07 | 36 ± 3 | 8 |
| NFLASH230 | 2020-10 | JUPITER | 38.2 ± 0.90 | 230.71 | 26.2 | 0.62 ± 0.05 | 0.76 ± 0.06 | 38 ± 3 | 16 |
| NFLASH230 | 2020-11 | MARS | 15.8 ± 1.48 | 230.71 | 26.2 | 0.57 ± 0.05 | 0.70 ± 0.06 | 41 ± 3 | 36 |
| NFLASH230 | 2020-09 | MARS | 21.9 ± 0.46 | 230.71 | 26.2 | 0.59 ± 0.05 | 0.73 ± 0.06 | 39 ± 3 | 8 |
| NFLASH230 | 2020-10 | MARS | 21.8 ± 0.91 | 230.71 | 26.2 | 0.59 ± 0.05 | 0.72 ± 0.06 | 40 ± 3 | 20 |
| NFLASH230 | 2020-09 | JUPITER | 41.6 ± 0.91 | 230.71 | 26.2 | 0.61 ± 0.05 | 0.75 ± 0.06 | 38 ± 3 | 16 |
| NFLASH230 | 2020-12 | MARS | 12.9 ± 1.04 | 230.71 | 26.2 | 0.56 ± 0.05 | 0.68 ± 0.06 | 42 ± 3 | 60 |
| PI230 | 2020-09 | MARS | 21.4 ± 0.01 | 242.54 | 25.0 | 0.58 ± 0.05 | 0.71 ± 0.06 | 40 ± 3 | 8 |
| SEPIA345 | 2020-10 | JUPITER | 39.4 ± 0.98 | 278.83 | 21.7 | 0.60 ± 0.05 | 0.74 ± 0.06 | 39 ± 3 | 16 |
| SEPIA345 | 2020-10 | MARS | 21.3 ± 0.86 | 278.83 | 21.7 | 0.65 ± 0.05 | 0.79 ± 0.06 | 36 ± 3 | 52 |
| SEPIA345 | 2020-11 | MARS | 19.8 ± 0.13 | 278.83 | 21.7 | 0.62 ± 0.05 | 0.76 ± 0.06 | 37 ± 3 | 12 |
| SEPIA345 | 2020-10 | JUPITER | 39.4 ± 0.98 | 291.17 | 20.8 | 0.60 ± 0.05 | 0.74 ± 0.06 | 39 ± 3 | 16 |
| SEPIA345 | 2020-10 | MARS | 21.3 ± 0.86 | 291.17 | 20.8 | 0.65 ± 0.05 | 0.80 ± 0.06 | 36 ± 3 | 52 |
| SEPIA345 | 2020-11 | MARS | 19.8 ± 0.13 | 291.17 | 20.8 | 0.64 ± 0.05 | 0.78 ± 0.06 | 36 ± 3 | 12 |
| LASMA345 | 2020-11 | MARS | 16.5 ± 0.16 | 332.27 | 18.2 | 0.58 ± 0.05 | 0.71 ± 0.06 | 40 ± 3 | 12 |
| LASMA345 | 2020-11 | URANUS | 3.7 ± 0.00 | 333.80 | 18.1 | 0.57 ± 0.10 | 0.70 ± 0.13 | 42 ± 8 | 8 |
| LASMA345 | 2020-09 | MARS | 21.7 ± 0.01 | 333.80 | 18.1 | 0.60 ± 0.05 | 0.73 ± 0.06 | 39 ± 3 | 8 |
| LASMA345 | 2020-11 | MARS | 18.2 ± 1.06 | 333.80 | 18.1 | 0.60 ± 0.05 | 0.74 ± 0.06 | 39 ± 3 | 20 |
| LASMA345 | 2020-11 | MARS | 16.5 ± 0.16 | 344.27 | 17.6 | 0.57 ± 0.05 | 0.70 ± 0.06 | 41 ± 3 | 12 |
| LASMA345 | 2020-11 | MARS | 18.2 ± 1.06 | 345.80 | 17.5 | 0.59 ± 0.05 | 0.73 ± 0.06 | 39 ± 3 | 20 |
| LASMA345 | 2020-11 | URANUS | 3.7 ± 0.00 | 345.80 | 17.5 | 0.55 ± 0.07 | 0.67 ± 0.09 | 44 ± 6 | 8 |
| LASMA345 | 2020-09 | MARS | 21.7 ± 0.01 | 345.80 | 17.5 | 0.64 ± 0.05 | 0.78 ± 0.07 | 37 ± 3 | 8 |
| SEPIA660 | 2020-09 | MARS | 21.3 ± 0.01 | 672.83 | 8.8 | 0.44 ± 0.03 | 0.52 ± 0.03 | 53 ± 3 | 12 |
| SEPIA660 | 2020-09 | JUPITER | 42.2 ± 0.00 | 672.83 | 8.8 | 0.42 ± 0.03 | 0.50 ± 0.04 | 55 ± 4 | 8 |
| SEPIA660 | 2020-09 | MARS | 21.3 ± 0.01 | 685.17 | 8.6 | 0.43 ± 0.03 | 0.51 ± 0.03 | 54 ± 4 | 12 |
| SEPIA660 | 2020-09 | JUPITER | 42.2 ± 0.00 | 685.17 | 8.6 | 0.42 ± 0.03 | 0.49 ± 0.04 | 55 ± 4 | 8 |
Note 1: The planet sizes (θS) and fluxes from the planet models usually have < 5% uncertanties. The values given in the table above are the average planet size from selected scans (at least 5 scans at a given frequency; for data older than 2018, a minimum of 3 scans is used instead since there was no systematic observations of antenna efficiencies back then). If the planet size is smaller than 0.4 x HPBW (θmb) then θS, aperture efficiency (ηa) and the point source sensitivity (Jy/K) will be highlighted since the correction [1-exp(-Q)]/Q (with Q=ln(2)(θS/θmb)²) for non-pointlike source is < 5%.
Note 2: The aperture efficiency (ηa) intrinsicly includes the effects due to error beams, blockage, spillover, etc., because it is measured on sky toward a planet. Thus, it is lower than the expected aperture efficiency due exclusively to the surface accuracy as described by the original Ruze equation.
Note 3: Although the correction for the influence of the source size mentioned in Note 1 is only a first order correction (i.e, it asumes a clean Gaussian beam and does not take into account the effect of the sidelobes nor the error beams), we can consider our estimates a good approximation of the actual main beam efficiency (ηmb).
The spectra delivered by the APEX real-time calibration software (Muders et al. 2006, A&A, 454, L25) is in GILDAS/CLASS format (Pety J., 2005, in SF2A-2005, p. 721) in the corrected (for atmospheric attenuation, the forward efficiency and signal band gain) antenna temperature TA* scale (Ulich & Haas, 1976, ApJ, Suppl. Ser., Vol. 30, p. 247). The forward efficiency (ηfw) in the header is set to the actual value (<1.0). The beam efficiency (ηmb) is set to 1.0. In order to switch to the TMB scale, one needs to multiply the data by ηfw/ηmb. Any further modification of ηmb after this switch of scale needs a multiplication by ηmb_old/ηmb_new. The "MODIFY BEAM_EFF" command in CLASS does the latter, i.e. it will use the existing eta_beam (=BEAM_EFF) as ηmb_old and the supplied value as ηmb_new. That is why the first step to switch to TMB needs an initial BEAM_EFF set to FORWARD_EFF. Hence, for conversion of APEX data to main beam brightness temperature TMB scale, we recommend using this CLASS script, after loading the data and getting a scan in the CLASS environment.
For point-like (relative to the beam size) science targets we recommend to use the ηmb values estimated from observations towards Uranus or Mars (when smaller than 0.4 x HPBW). For semi-extended sources (not point-like but unresolved by the beam) values estimated from Mars (when 0.4 x HPBW < θS < HPBW) are better. For extended sources (resolved by the beam) the values estimated from Jupiter or Mars (when larger than the beam) are recommended.
Note 4: Because of the new sub-reflector the forward efficiency (ηf=0.95) for 2018+ have smaller uncertainty of about 5% in contrast to the 10% uncertainty considered for previous years. Other efficiencies (ηa, ηmb and Jy/K) include the uncertainties of: the forward efficiency, the planet model (4%), the beam size (5%) and the uncertainty of the observed peak brightness temperature (usually < 10% for Uranus, and < 5% for Mars and Jupiter).
Note 5: The Jansky to Kelvin conversion factor for the APEX antenna can also be approximated by
ηf24.4/ηa (Jy/K).
According to the Ruze formula the aperture efficiency (ηa) and, hence, the main beam efficiency (ηmb), will
degrade with shorter wavelength, λ, as
ηa (λ) = ηa (∞) exp(-16π²σ²/λ²)
where σ is the antenna surface RMS value (i.e. describing
small scale deviations from a perfect surface).
Likewise, according to Downes (1989) the main beam efficiency can be estimated from the aperture efficiency as
ηmb (λ) = 0.8899 [θmb/(λ/D)]² ηa
with θmb ≈ k λ/D (assuming a Gaussian beam) where k is a factor that depends on the illumination taper
and blockage of the aperture (e.g., Christiansen & Högbom 1985; Kraus 1986). For most APEX receivers we found k ≈ 1.17 which
correspond to an edge tapering of about 13.9 dB (consistent with the value assumed by Güsten et al. 2006). Thus, the main beam efficiency can be
approximated as: ηmb (λ) = 0.8899 (1.17)² ηa.
From the 2018 commissioning we recommend using θmb ≈ (9.0 ± 0.1) 658/fGHz for the full frequency range of SEPIA660.
For the estimates we select only well focused and calibrated pointing scans toward Mars, Jupiter and Uranus (when available; during most of 2017 Mars was in sun avoidance). Pointing scans with offsets smaller than 10% of the beam size are selected for the estimates. Only the cross-scan in Azimuth are used and we discard the scans where the FWHM from the Azimuth and Elevation cross-scans differ by more than 10%. The aparent size and fluxes of the planets for a particular date and frequency are obtained from the corresponding models implemented in CASA ( CASA Guides: Solar System Models; ALMA memo #594). Comparing with other existing models (e.g., in GILDAS/ASTRO and in the work by Lellouch et al., 2006) we found they agree within 15%. Details of the theory and calculations used in our estimates can be found here. For a more elaborated explanation of the formulas we refer to the IRAM 30m internal documents cali_rep.pdf or spatial_response_framework_v1.8.pdf.
Efficiencies for the PI CHAMP+ instrument can be found at the corresponding web page at MPIfR.
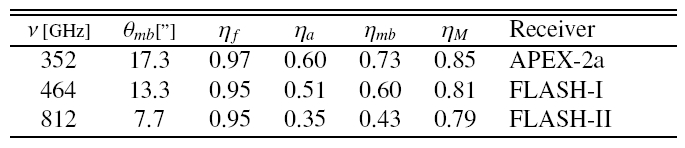
The historical table below was adapted from Güsten et al. (2006, A&A 454, L13-L16). Beam sizes (θmb) were determined by deconvolving planet scans. The listed efficiencies are forward efficiency (ηf), aperture efficiency (ηa), main beam efficiency (ηmb), and Moon efficiency (ηM). The uncertainties of the listed efficiencies are expected to be 5-10%. For comparison with older values you can still find the former table of antenna efficiencies here.